Axial tilt

Last updated

The positive pole of a planet is defined by the right-hand rule: if the fingers of the right hand are curled in the direction of the rotation then the thumb points to the positive pole. The axial tilt is defined as the angle between the direction of the positive pole and the normal to the orbital plane. The angles for Earth, Uranus, and Venus are approximately 23deg, 97deg, and 177deg respectively. Planet axis comparison.png
The positive pole of a planet is defined by the right-hand rule: if the fingers of the right hand are curled in the direction of the rotation then the thumb points to the positive pole. The axial tilt is defined as the angle between the direction of the positive pole and the normal to the orbital plane. The angles for Earth, Uranus, and Venus are approximately 23°, 97°, and 177° respectively.

In astronomy, axial tilt, also known as obliquity, is the angle between an object's rotational axis and its orbital axis, which is the line perpendicular to its orbital plane; equivalently, it is the angle between its equatorial plane and orbital plane. [1] It differs from orbital inclination.

Contents

At an obliquity of 0 degrees, the two axes point in the same direction; that is, the rotational axis is perpendicular to the orbital plane.

The rotational axis of Earth, for example, is the imaginary line that passes through both the North Pole and South Pole, whereas the Earth's orbital axis is the line perpendicular to the imaginary plane through which the Earth moves as it revolves around the Sun; the Earth's obliquity or axial tilt is the angle between these two lines.

Over the course of an orbital period, the obliquity usually does not change considerably, and the orientation of the axis remains the same relative to the background of stars. This causes one pole to be pointed more toward the Sun on one side of the orbit, and more away from the Sun on the other side—the cause of the seasons on Earth.

Standards

There are two standard methods of specifying a planet's tilt. One way is based on the planet's north pole, defined in relation to the direction of Earth's north pole, and the other way is based on the planet's positive pole, defined by the right-hand rule:

Earth

Earth's orbital plane is known as the ecliptic plane, and Earth's tilt is known to astronomers as the obliquity of the ecliptic, being the angle between the ecliptic and the celestial equator on the celestial sphere. [6] It is denoted by the Greek letter Epsilon ε .

Earth currently has an axial tilt of about 23.44°. [7] This value remains about the same relative to a stationary orbital plane throughout the cycles of axial precession. [8] But the ecliptic (i.e., Earth's orbit) moves due to planetary perturbations, and the obliquity of the ecliptic is not a fixed quantity. At present, it is decreasing at a rate of about 46.8″ [9] per century (see details in Short term below).

History

The ancient Greeks had good measurements of the obliquity since about 350 BCE, when Pytheas of Marseilles measured the shadow of a gnomon at the summer solstice. [10] About 830 CE, the Caliph Al-Mamun of Baghdad directed his astronomers to measure the obliquity, and the result was used in the Arab world for many years. [11] In 1437, Ulugh Beg determined the Earth's axial tilt as 23°30′17″ (23.5047°). [12]

During the Middle Ages, it was widely believed that both precession and Earth's obliquity oscillated around a mean value, with a period of 672 years, an idea known as trepidation of the equinoxes. Perhaps the first to realize this was incorrect (during historic time) was Ibn al-Shatir in the fourteenth century [13] and the first to realize that the obliquity is decreasing at a relatively constant rate was Fracastoro in 1538. [14] The first accurate, modern, western observations of the obliquity were probably those of Tycho Brahe from Denmark, about 1584, [15] although observations by several others, including al-Ma'mun, al-Tusi, [16] Purbach, Regiomontanus, and Walther, could have provided similar information.

Seasons

The axis of Earth remains oriented in the same direction with reference to the background stars regardless of where it is in its orbit. Northern hemisphere summer occurs at the right side of this diagram, where the north pole (red) is directed toward the Sun, winter at the left. Earth tilt animation.gif
The axis of Earth remains oriented in the same direction with reference to the background stars regardless of where it is in its orbit. Northern hemisphere summer occurs at the right side of this diagram, where the north pole (red) is directed toward the Sun, winter at the left.

Earth's axis remains tilted in the same direction with reference to the background stars throughout a year (regardless of where it is in its orbit) due to the gyroscope effect. This means that one pole (and the associated hemisphere of Earth) will be directed away from the Sun at one side of the orbit, and half an orbit later (half a year later) this pole will be directed towards the Sun. This is the cause of Earth's seasons. Summer occurs in the Northern hemisphere when the north pole is directed toward the Sun. Variations in Earth's axial tilt can influence the seasons and is likely a factor in long-term climatic change (also see Milankovitch cycles).

Relationship between Earth's axial tilt (e) to the tropical and polar circles Axial tilt vs tropical and polar circles.svg
Relationship between Earth's axial tilt (ε) to the tropical and polar circles

Oscillation

Short term

Obliquity of the ecliptic for 20,000 years, from Laskar (1986). The red point represents the year 2000. Obliquity of the ecliptic laskar.PNG
Obliquity of the ecliptic for 20,000 years, from Laskar (1986). The red point represents the year 2000.

The exact angular value of the obliquity is found by observation of the motions of Earth and planets over many years. Astronomers produce new fundamental ephemerides as the accuracy of observation improves and as the understanding of the dynamics increases, and from these ephemerides various astronomical values, including the obliquity, are derived.

Annual almanacs are published listing the derived values and methods of use. Until 1983, the Astronomical Almanac's angular value of the mean obliquity for any date was calculated based on the work of Newcomb, who analyzed positions of the planets until about 1895:

ε = 23°27′8.26″ − 46.845″ T − 0.0059″ T2 + 0.00181″ T3

where ε is the obliquity and T is tropical centuries from B1900.0 to the date in question. [17]

From 1984, the Jet Propulsion Laboratory's DE series of computer-generated ephemerides took over as the fundamental ephemeris of the Astronomical Almanac. Obliquity based on DE200, which analyzed observations from 1911 to 1979, was calculated:

ε = 23°26′21.448″ − 46.8150″ T − 0.00059″ T2 + 0.001813″ T3

where hereafter T is Julian centuries from J2000.0. [18]

JPL's fundamental ephemerides have been continually updated. For instance, according to IAU resolution in 2006 in favor of the P03 astronomical model, the Astronomical Almanac for 2010 specifies: [19]

ε = 23°26′21.406″ − 46.836769″ T − 0.0001831″ T2 + 0.00200340″ T3 − 5.76″ × 10−7T4 − 4.34″ × 10−8T5

These expressions for the obliquity are intended for high precision over a relatively short time span, perhaps ± several centuries. [20] Jacques Laskar computed an expression to order T10 good to 0.02″ over 1000 years and several arcseconds over 10,000 years.

ε = 23°26′21.448″ − 4680.93″ t − 1.55″ t2 + 1999.25″ t3 − 51.38″ t4 − 249.67″ t5 − 39.05″ t6 + 7.12″ t7 + 27.87″ t8 + 5.79″ t9 + 2.45″ t10

where here t is multiples of 10,000 Julian years from J2000.0. [21]

These expressions are for the so-called mean obliquity, that is, the obliquity free from short-term variations. Periodic motions of the Moon and of Earth in its orbit cause much smaller (9.2 arcseconds) short-period (about 18.6 years) oscillations of the rotation axis of Earth, known as nutation, which add a periodic component to Earth's obliquity. [22] [23] The true or instantaneous obliquity includes this nutation. [24]

Long term

Using numerical methods to simulate Solar System behavior over a period of several million years, long-term changes in Earth's orbit, and hence its obliquity, have been investigated. For the past 5 million years, Earth's obliquity has varied between 22°2′33″ and 24°30′16″, with a mean period of 41,040 years. This cycle is a combination of precession and the largest term in the motion of the ecliptic. For the next 1 million years, the cycle will carry the obliquity between 22°13′44″ and 24°20′50″. [25]

The Moon has a stabilizing effect on Earth's obliquity. Frequency map analysis conducted in 1993 suggested that, in the absence of the Moon, the obliquity could change rapidly due to orbital resonances and chaotic behavior of the Solar System, reaching as high as 90° in as little as a few million years (also see Orbit of the Moon ). [26] [27] However, more recent numerical simulations [28] made in 2011 indicated that even in the absence of the Moon, Earth's obliquity might not be quite so unstable; varying only by about 20–25°. To resolve this contradiction, diffusion rate of obliquity has been calculated, and it was found that it takes more than billions of years for Earth's obliquity to reach near 90°. [29] The Moon's stabilizing effect will continue for less than two billion years. As the Moon continues to recede from Earth due to tidal acceleration, resonances may occur which will cause large oscillations of the obliquity. [30]

Obliquity berger -5000000 to 0.png
Obliquity berger 0 to 1000000.png
Long-term obliquity of the ecliptic. Left: for the past 5 million years; the obliquity varies only from about 22.0° to 24.5°. Right: for the next 1 million years; note the approx. 41,000-year period of variation. In both graphs, the red point represents the year 1850. [31]

Solar System bodies

Axial tilt of eight planets and two dwarf planets, Ceres and Pluto

All four of the innermost, rocky planets of the Solar System may have had large variations of their obliquity in the past. Since obliquity is the angle between the axis of rotation and the direction perpendicular to the orbital plane, it changes as the orbital plane changes due to the influence of other planets. But the axis of rotation can also move (axial precession), due to torque exerted by the Sun on a planet's equatorial bulge. Like Earth, all of the rocky planets show axial precession. If the precession rate were very fast the obliquity would actually remain fairly constant even as the orbital plane changes. [32] The rate varies due to tidal dissipation and core-mantle interaction, among other things. When a planet's precession rate approaches certain values, orbital resonances may cause large changes in obliquity. The amplitude of the contribution having one of the resonant rates is divided by the difference between the resonant rate and the precession rate, so it becomes large when the two are similar. [32]

Mercury and Venus have most likely been stabilized by the tidal dissipation of the Sun. Earth was stabilized by the Moon, as mentioned above, but before its formation, Earth, too, could have passed through times of instability. Mars's obliquity is quite variable over millions of years and may be in a chaotic state; it varies as much as 0° to 60° over some millions of years, depending on perturbations of the planets. [26] [33] Some authors dispute that Mars's obliquity is chaotic, and show that tidal dissipation and viscous core-mantle coupling are adequate for it to have reached a fully damped state, similar to Mercury and Venus. [3] [34]

The occasional shifts in the axial tilt of Mars have been suggested as an explanation for the appearance and disappearance of rivers and lakes over the course of the existence of Mars. A shift could cause a burst of methane into the atmosphere, causing warming, but then the methane would be destroyed and the climate would become arid again. [35] [36]

The obliquities of the outer planets are considered relatively stable.

Axis and rotation of selected Solar System bodies
Body NASA, J2000.0 [37] epoch IAU, 0h 0 January 2010 TT [38] epoch
Axial tilt
(degrees)		North PoleRotational
period
(hours)		Axial tilt
(degrees)		North PoleRotation
(deg./day)
R.A. (degrees) Dec. (degrees) R.A. (degrees) Dec. (degrees)
Sun 7.25286.1363.87609.12 [A] 7.25 [B] 286.1563.8914.18
Mercury 0.03281.0161.411407.60.01281.0161.456.14
Venus 2.64272.7667.16−5832.62.64272.7667.16−1.48
Earth 23.440.0090.0023.9323.44Undefined90.00360.99
Moon 6.68––655.731.54 [C] 270.0066.5413.18
Mars 25.19317.6852.8924.6225.19317.6752.88350.89
Jupiter 3.13268.0664.509.93 [D] 3.12268.0664.50870.54 [D]
Saturn 26.7340.5983.5410.66 [D] 26.7340.5983.54810.79 [D]
Uranus 82.23257.31−15.18−17.24 [D] 82.23257.31−15.18−501.16 [D]
Neptune 28.32299.3342.9516.11 [D] 28.33299.4042.95536.31 [D]
Pluto [E] 57.47312.99 [E] 6.16 [E] −153.2960.41312.996.16−56.36
  1. ↑ At 16° latitude; the Sun's rotation varies with latitude.
  2. ↑ With respect to the ecliptic of 1850.
  3. ↑ With respect to the ecliptic; the Moon's orbit is inclined 5.16° to the ecliptic.
  4. 1 2 3 4 5 6 7 8 From the origin of the radio emissions; the visible clouds generally rotate at different rate.
  5. 1 2 3 NASA lists the coordinates of Pluto's positive pole; noted values have been reinterpreted to correspond to the north/negative pole.

Extrasolar planets

The stellar obliquity ψs, i.e. the axial tilt of a star with respect to the orbital plane of one of its planets, has been determined for only a few systems. By 2012, 49 stars have had sky-projected spin-orbit misalignment λ has been observed, [39] which serves as a lower limit to ψs. Most of these measurements rely on the Rossiter–McLaughlin effect. Since the launch of space-based telescopes such as Kepler space telescope, it has been made possible to determine and estimate the obliquity of an extrasolar planet. The rotational flattening of the planet and the entourage of moons and/or rings, which are traceable with high-precision photometry provide access to planetary obliquity, ψp. Many extrasolar planets have since had their obliquity determined, such as Kepler-186f and Kepler-413b. [40] [41]

Astrophysicists have applied tidal theories to predict the obliquity of extrasolar planets. It has been shown that the obliquities of exoplanets in the habitable zone around low-mass stars tend to be eroded in less than 109 years, [42] [43] which means that they would not have tilt-induced seasons as Earth has.

See also

Related Research Articles

<span class="mw-page-title-main">Ecliptic</span> Apparent path of the Sun on the celestial sphere

The ecliptic or ecliptic plane is the orbital plane of Earth around the Sun. From the perspective of an observer on Earth, the Sun's movement around the celestial sphere over the course of a year traces out a path along the ecliptic against the background of stars. The ecliptic is an important reference plane and is the basis of the ecliptic coordinate system.

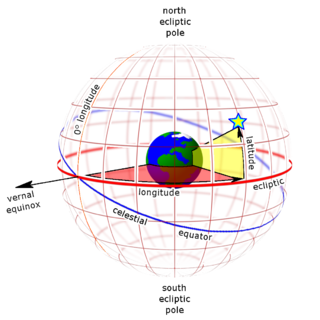
<span class="mw-page-title-main">Ecliptic coordinate system</span> Celestial coordinate system used to describe Solar System objects

In astronomy, the ecliptic coordinate system is a celestial coordinate system commonly used for representing the apparent positions, orbits, and pole orientations of Solar System objects. Because most planets and many small Solar System bodies have orbits with only slight inclinations to the ecliptic, using it as the fundamental plane is convenient. The system's origin can be the center of either the Sun or Earth, its primary direction is towards the March equinox, and it has a right-hand convention. It may be implemented in spherical or rectangular coordinates.

<span class="mw-page-title-main">Orbital inclination</span> Angle between a reference plane and the plane of an orbit

Orbital inclination measures the tilt of an object's orbit around a celestial body. It is expressed as the angle between a reference plane and the orbital plane or axis of direction of the orbiting object.

<span class="mw-page-title-main">Axial precession</span> Change of rotational axis in an astronomical body

In astronomy, axial precession is a gravity-induced, slow, and continuous change in the orientation of an astronomical body's rotational axis. In the absence of precession, the astronomical body's orbit would show axial parallelism. In particular, axial precession can refer to the gradual shift in the orientation of Earth's axis of rotation in a cycle of approximately 26,000 years. This is similar to the precession of a spinning top, with the axis tracing out a pair of cones joined at their apices. The term "precession" typically refers only to this largest part of the motion; other changes in the alignment of Earth's axis—nutation and polar motion—are much smaller in magnitude.

<span class="mw-page-title-main">Solar time</span> Calculation of elapsed time by the apparent position of the sun

Solar time is a calculation of the passage of time based on the position of the Sun in the sky. The fundamental unit of solar time is the day, based on the synodic rotation period. Traditionally, there are three types of time reckoning based on astronomical observations: apparent solar time and mean solar time, and sidereal time, which is based on the apparent motions of stars other than the Sun.

<span class="mw-page-title-main">Celestial equator</span> Projection of Earths equator out into space

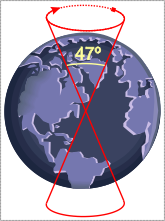
The celestial equator is the great circle of the imaginary celestial sphere on the same plane as the equator of Earth. By extension, it is also a plane of reference in the equatorial coordinate system. In other words, the celestial equator is an abstract projection of the terrestrial equator into outer space. Due to Earth's axial tilt, the celestial equator is currently inclined by about 23.44° with respect to the ecliptic, but has varied from about 22.0° to 24.5° over the past 5 million years due to perturbation from other planets.

<span class="mw-page-title-main">Lunar precession</span> Changes in the moons rotation and orbit

Lunar precession is a term used for three different precession motions related to the Moon. First, it can refer to change in orientation of the lunar rotational axis with respect to a reference plane, following the normal rules of precession followed by spinning objects. In addition, the orbit of the Moon undergoes two important types of precessional motion: apsidal and nodal.

<span class="mw-page-title-main">Libration</span> Apparent oscillation of a minor body seen from the major body it orbits

In lunar astronomy, libration is the cyclic variation in the apparent position of the Moon perceived by Earth-bound observers and caused by changes between the orbital and rotational planes of the moon. It causes an observer to see slightly different hemispheres of the surface at different times. It is similar in both cause and effect to the changes in the Moon's apparent size due to changes in distance. It is caused by three mechanisms detailed below, two of which cause a relatively tiny physical libration via tidal forces exerted by the Earth. Such true librations are known as well for other moons with locked rotation.

<span class="mw-page-title-main">Milankovitch cycles</span> Global climate cycles

Milankovitch cycles describe the collective effects of changes in the Earth's movements on its climate over thousands of years. The term was coined and named after the Serbian geophysicist and astronomer Milutin Milanković. In the 1920s, he hypothesized that variations in eccentricity, axial tilt, and precession combined to result in cyclical variations in the intra-annual and latitudinal distribution of solar radiation at the Earth's surface, and that this orbital forcing strongly influenced the Earth's climatic patterns.

<span class="mw-page-title-main">Earth's orbit</span> Trajectory of Earth around the Sun

Earth orbits the Sun at an average distance of 149.60 million km (92.96 million mi), or 8.317 light-minutes, in a counterclockwise direction as viewed from above the Northern Hemisphere. One complete orbit takes 365.256 days, during which time Earth has traveled 940 million km (584 million mi). Ignoring the influence of other Solar System bodies, Earth's orbit, also called Earth's revolution, is an ellipse with the Earth–Sun barycenter as one focus with a current eccentricity of 0.0167. Since this value is close to zero, the center of the orbit is relatively close to the center of the Sun.

<span class="mw-page-title-main">Great Year</span> Length of time

The term Great Year has more than one major meaning. It is defined by scientific astronomy as "The period of one complete cycle of the equinoxes around the ecliptic, or about 25,800 years". Ptolemy reported that his teacher Hipparchus, by comparing the position of the vernal equinox against the fixed stars in his time and in earlier observations, discovered that it shifts westward approximately one degree every 72 years. Thus the time it would take the equinox to make a complete revolution through all the zodiac constellations and return to its original position would be approximately 25,920 years. In the heliocentric model, the precession can be pictured as the axis of the Earth's rotation making a slow revolution around the normal to the plane of the ecliptic. The position of the Earth's axis in the northern night sky currently almost aligns with the star Polaris, the North Star. But as the direction of the axis is changing, this is a passing coincidence which was not always so and will not be so again until a Great Year has passed.

<span class="mw-page-title-main">Astronomy on Mars</span> Astronomical phenomena viewed from the planet Mars

Many astronomical phenomena viewed from the planet Mars are the same as or similar to those seen from Earth; but some are quite different. For example, because the atmosphere of Mars does not contain an ozone layer, it is also possible to make UV observations from the surface of Mars.

Cassini's laws provide a compact description of the motion of the Moon. They were established in 1693 by Giovanni Domenico Cassini, a prominent scientist of his time.

<span class="mw-page-title-main">Orbit of the Moon</span> The Moons circuit around Earth

The Moon orbits Earth in the prograde direction and completes one revolution relative to the Vernal Equinox and the stars in about 27.32 days and one revolution relative to the Sun in about 29.53 days. Earth and the Moon orbit about their barycentre, which lies about 4,670 km from Earth's centre, forming a satellite system called the Earth–Moon system. On average, the distance to the Moon is about 384,400 km (238,900 mi) from Earth's centre, which corresponds to about 60 Earth radii or 1.282 light-seconds.

<span class="mw-page-title-main">Habitability of natural satellites</span> Measure of the potential of natural satellites to have environments hospitable to life

The habitability of natural satellites is the potential of moons to provide habitats for life, though it is not an indicator that they harbor it. Natural satellites are expected to outnumber planets by a large margin and the study of their habitability is therefore important to astrobiology and the search for extraterrestrial life. There are, nevertheless, significant environmental variables specific to moons.

<span class="mw-page-title-main">Apsidal precession</span> Rotation of a celestial bodys orbital line of apsides

In celestial mechanics, apsidal precession is the precession of the line connecting the apsides of an astronomical body's orbit. The apsides are the orbital points farthest (apoapsis) and closest (periapsis) from its primary body. The apsidal precession is the first time derivative of the argument of periapsis, one of the six main orbital elements of an orbit. Apsidal precession is considered positive when the orbit's axis rotates in the same direction as the orbital motion. An apsidal period is the time interval required for an orbit to precess through 360°, which takes the Earth about 112,000 years and the Moon about 8.85 years.

<span class="mw-page-title-main">Retrograde and prograde motion</span> Relative directions of orbit or rotation

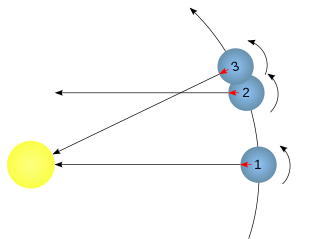
Retrograde motion in astronomy is, in general, orbital or rotational motion of an object in the direction opposite the rotation of its primary, that is, the central object. It may also describe other motions such as precession or nutation of an object's rotational axis. Prograde or direct motion is more normal motion in the same direction as the primary rotates. However, "retrograde" and "prograde" can also refer to an object other than the primary if so described. The direction of rotation is determined by an inertial frame of reference, such as distant fixed stars.

This glossary of astronomy is a list of definitions of terms and concepts relevant to astronomy and cosmology, their sub-disciplines, and related fields. Astronomy is concerned with the study of celestial objects and phenomena that originate outside the atmosphere of Earth. The field of astronomy features an extensive vocabulary and a significant amount of jargon.

Astronomical nutation is a phenomenon which causes the orientation of the axis of rotation of a spinning astronomical object to vary over time. It is caused by the gravitational forces of other nearby bodies acting upon the spinning object. Although they are caused by the same effect operating over different timescales, astronomers usually make a distinction between precession, which is a steady long-term change in the axis of rotation, and nutation, which is the combined effect of similar shorter-term variations.

<span class="mw-page-title-main">Axial parallelism</span> Characteristic of a spinning body in space

Axial parallelism is the characteristic of a rotating body in which the direction of the axis of rotation remains fixed as the object moves through space. In astronomy, this characteristic is found in astronomical bodies in orbit. It is the same effect that causes a gyroscope's axis of rotation to remain constant as Earth rotates, allowing the devices to measure Earth's rotation.

References

  1. ↑ U.S. Naval Observatory Nautical Almanac Office (1992). P. Kenneth Seidelmann (ed.). Explanatory Supplement to the Astronomical Almanac. University Science Books. p. 733. ISBN   978-0-935702-68-2.
  2. ↑ Explanatory Supplement 1992, p. 384
  3. 1 2 Correia, Alexandre C. M.; Laskar, Jacques; de Surgy, Olivier Néron (May 2003). "Long-term evolution of the spin of Venus I. theory" (PDF). Icarus. 163 (1): 1–23. Bibcode:2003Icar..163....1C. doi:10.1016/S0019-1035(03)00042-3. Archived (PDF) from the original on 9 October 2022.
  4. ↑ Correia, A. C. M.; Laskar, J. (2003). "Long-term evolution of the spin of Venus: II. numerical simulations" (PDF). Icarus. 163 (1): 24–45. Bibcode:2003Icar..163...24C. doi:10.1016/S0019-1035(03)00043-5. Archived (PDF) from the original on 9 October 2022.
  5. ↑ Seidelmann, P. Kenneth; Archinal, B. A.; a'Hearn, M. F.; Conrad, A.; Consolmagno, G. J.; Hestroffer, D.; Hilton, J. L.; Krasinsky, G. A.; Neumann, G.; Oberst, J.; Stooke, P.; Tedesco, E. F.; Tholen, D. J.; Thomas, P. C.; Williams, I. P. (2007). "Report of the IAU/IAG Working Group on cartographic coordinates and rotational elements: 2006". Celestial Mechanics and Dynamical Astronomy. 98 (3): 155–180. Bibcode:2007CeMDA..98..155S. doi: 10.1007/s10569-007-9072-y .
  6. ↑ U.S. Naval Observatory Nautical Almanac Office; U.K. Hydrographic Office; H.M. Nautical Almanac Office (2008). The Astronomical Almanac for the Year 2010. US Government Printing Office. p. M11. ISBN   978-0-7077-4082-9.
  7. ↑ "Glossary" in Astronomical Almanac Online. (2023). Washington DC: United States Naval Observatory. s.v. obliquity.
  8. ↑ Chauvenet, William (1906). A Manual of Spherical and Practical Astronomy. Vol. 1. J. B. Lippincott. pp. 604–605.
  9. ↑ Ray, Richard D.; Erofeeva, Svetlana Y. (4 February 2014). "Long-period tidal variations in the length of day". Journal of Geophysical Research: Solid Earth. 119 (2): 1498–1509. Bibcode:2014JGRB..119.1498R. doi: 10.1002/2013JB010830 .
  10. ↑ Gore, J. E. (1907). Astronomical Essays Historical and Descriptive. Chatto & Windus. p.  61.
  11. ↑ Marmery, J. V. (1895). Progress of Science. Chapman and Hall, ld. p.  33.
  12. ↑ Sédillot, L.P.E.A. (1853). Prolégomènes des tables astronomiques d'OlougBeg: Traduction et commentaire. Paris: Firmin Didot Frères. pp. 87 & 253.
  13. ↑ Saliba, George (1994). A History of Arabic Astronomy: Planetary Theories During the Golden Age of Islam. p. 235.
  14. ↑ Dreyer, J. L. E. (1890). Tycho Brahe. A. & C. Black. p.  355.
  15. ↑ Dreyer (1890), p. 123
  16. ↑ Sayili, Aydin (1981). The Observatory in Islam. p. 78.
  17. ↑ U.S. Naval Observatory Nautical Almanac Office; H.M. Nautical Almanac Office (1961). Explanatory Supplement to the Astronomical Ephemeris and the American Ephemeris and Nautical Almanac. H.M. Stationery Office. Section 2B.
  18. ↑ U.S. Naval Observatory; H.M. Nautical Almanac Office (1989). The Astronomical Almanac for the Year 1990. US Government Printing Office. p. B18. ISBN   978-0-11-886934-8.
  19. ↑ Astronomical Almanac 2010, p. B52
  20. ↑ Newcomb, Simon (1906). A Compendium of Spherical Astronomy. MacMillan. pp.  226–227.
  21. ↑ See table 8 and eq. 35 in Laskar, J. (1986). "Secular terms of classical planetary theories using the results of general theory". Astronomy and Astrophysics. 157 (1): 59–70. Bibcode:1986A&A...157...59L. and erratum to article Laskar, J. (1986). "Erratum: Secular terms of classical planetary theories using the results of general theory". Astronomy and Astrophysics. 164: 437. Bibcode:1986A&A...164..437L. Units in article are arcseconds, which may be more convenient.
  22. ↑ Explanatory Supplement (1961), sec. 2C
  23. ↑ "Basics of Space Flight, Chapter 2". Jet Propulsion Laboratory/NASA. 29 October 2013. Retrieved 26 March 2015.
  24. ↑ Meeus, Jean (1991). "Chapter 21". Astronomical Algorithms. Willmann-Bell. ISBN   978-0-943396-35-4.
  25. ↑ Berger, A.L. (1976). "Obliquity and Precession for the Last 5000000 Years". Astronomy and Astrophysics . 51 (1): 127–135. Bibcode:1976A&A....51..127B.
  26. 1 2 Laskar, J.; Robutel, P. (1993). "The Chaotic Obliquity of the Planets" (PDF). Nature . 361 (6413): 608–612. Bibcode:1993Natur.361..608L. doi:10.1038/361608a0. S2CID   4372237. Archived from the original (PDF) on 23 November 2012.
  27. ↑ Laskar, J.; Joutel, F.; Robutel, P. (1993). "Stabilization of the Earth's Obliquity by the Moon" (PDF). Nature. 361 (6413): 615–617. Bibcode:1993Natur.361..615L. doi:10.1038/361615a0. S2CID   4233758. Archived (PDF) from the original on 9 October 2022.
  28. ↑ Lissauer, J.J.; Barnes, J.W.; Chambers, J.E. (2011). "Obliquity variations of a moonless Earth" (PDF). Icarus . 217 (1): 77–87. Bibcode:2012Icar..217...77L. doi:10.1016/j.icarus.2011.10.013. Archived (PDF) from the original on 8 June 2013.
  29. ↑ Li, Gongjie; Batygin, Konstantin (20 July 2014). "On the Spin-axis Dynamics of a Moonless Earth". Astrophysical Journal. 790 (1): 69–76. arXiv: 1404.7505 . Bibcode:2014ApJ...790...69L. doi:10.1088/0004-637X/790/1/69. S2CID   119295403.
  30. ↑ Ward, W.R. (1982). "Comments on the Long-Term Stability of the Earth's Obliquity". Icarus . 50 (2–3): 444–448. Bibcode:1982Icar...50..444W. doi:10.1016/0019-1035(82)90134-8.
  31. ↑ Berger, 1976.
  32. 1 2 William Ward (20 July 1973). "Large-Scale Variations in the Obliquity of Mars". Science. 181 (4096): 260–262. Bibcode:1973Sci...181..260W. doi:10.1126/science.181.4096.260. PMID   17730940. S2CID   41231503.
  33. ↑ Touma, J.; Wisdom, J. (1993). "The Chaotic Obliquity of Mars" (PDF). Science . 259 (5099): 1294–1297. Bibcode:1993Sci...259.1294T. doi:10.1126/science.259.5099.1294. PMID   17732249. S2CID   42933021. Archived (PDF) from the original on 25 June 2010.
  34. ↑ Correia, Alexandre C.M; Laskar, Jacques (2009). "Mercury's capture into the 3/2 spin-orbit resonance including the effect of core-mantle friction". Icarus. 201 (1): 1–11. arXiv: 0901.1843 . Bibcode:2009Icar..201....1C. doi:10.1016/j.icarus.2008.12.034. S2CID   14778204.
  35. ↑ Rebecca Boyle (7 October 2017). "Methane burps on young Mars helped it keep its liquid water". New Scientist.
  36. ↑ Edwin Kite; et al. (2 October 2017). "Methane bursts as a trigger for intermittent lake-forming climates on post-Noachian Mars" (PDF). Nature Geoscience. 10 (10): 737–740. arXiv: 1611.01717 . Bibcode:2017NatGe..10..737K. doi:10.1038/ngeo3033. S2CID   102484593. Archived (PDF) from the original on 23 July 2018.
  37. ↑ Planetary Fact Sheets, at http://nssdc.gsfc.nasa.gov
  38. ↑ Astronomical Almanac 2010, pp. B52, C3, D2, E3, E55
  39. ↑ Heller, R. "Holt-Rossiter-McLaughlin Encyclopaedia". René Heller. Retrieved 24 February 2012.
  40. ↑ Grossman, David (29 June 2018). "Study Shows Exoplanet Has a Stable Axis Just Like Earth". Popular Mechanics. Retrieved 26 February 2024.
  41. ↑ "Kepler Finds a Very Wobbly Planet - NASA". 4 February 2014. Retrieved 26 February 2024.
  42. ↑ Heller, R.; Leconte, J.; Barnes, R. (2011). "Tidal obliquity evolution of potentially habitable planets". Astronomy and Astrophysics . 528: A27. arXiv: 1101.2156 . Bibcode:2011A&A...528A..27H. doi:10.1051/0004-6361/201015809. S2CID   118784209.
  43. ↑ Heller, R.; Leconte, J.; Barnes, R. (2011). "Habitability of Extrasolar Planets and Tidal Spin Evolution". Origins of Life and Evolution of Biospheres. 41 (6): 539–43. arXiv: 1108.4347 . Bibcode:2011OLEB...41..539H. doi:10.1007/s11084-011-9252-3. PMID   22139513. S2CID   10154158.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.