1.3 solving equations y
- 1. Equations Math 260 Dr. Frank Ma LA Harbor College
- 2. Summary for Solving Equations Equations The most important equations one has to know how to solve are the 1st and 2nd degree equations: * The solution of the 1st degree equation ax + b = 0 is x = –b/a * To solve the 2nd degree equation ax2 + bx + c = 0 i. Try the factoring method first. ii. Use the quadratic formula if it is not factorable: x = –b ± b2 – 4ac 2a To solve most other types of equations such as rational equations or radical equations by hand, one has to transform them to problems of solving 1st or 2nd degree equations.
- 3. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Equations
- 4. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. Equations
- 5. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. If we order x = 100 pizzas, then the cost is 8(100) + 10 = $810. Equations
- 6. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. If we order x = 100 pizzas, then the cost is 8(100) + 10 = $810. Expressions calculate future results. Equations
- 7. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. If we order x = 100 pizzas, then the cost is 8(100) + 10 = $810. Expressions calculate future results. Now if we know the cost is $810 but forgot how many pizzas were ordered, we may backtrack to find x: Equations
- 8. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. If we order x = 100 pizzas, then the cost is 8(100) + 10 = $810. Expressions calculate future results. Now if we know the cost is $810 but forgot how many pizzas were ordered, we may backtrack to find x: 8x + 10 = 810 Equations
- 9. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. If we order x = 100 pizzas, then the cost is 8(100) + 10 = $810. Expressions calculate future results. Now if we know the cost is $810 but forgot how many pizzas were ordered, we may backtrack to find x: 8x + 10 = 810 8x = 800 –10 –10 subtract the delivery cost, so the pizzas cost $800, Equations
- 10. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. If we order x = 100 pizzas, then the cost is 8(100) + 10 = $810. Expressions calculate future results. Now if we know the cost is $810 but forgot how many pizzas were ordered, we may backtrack to find x: 8x + 10 = 810 8x = 800 –10 –10 subtract the delivery cost, so the pizzas cost $800, divide this by $8/per pizza, x = 100 (pizzas) 8 8 Equations
- 11. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. If we order x = 100 pizzas, then the cost is 8(100) + 10 = $810. Expressions calculate future results. Now if we know the cost is $810 but forgot how many pizzas were ordered, we may backtrack to find x: 8x + 10 = 810 8x = 800 –10 –10 subtract the delivery cost, so the pizzas cost $800, divide this by $8/per pizza, x = 100 8 8 “8x + 10 = 810” is called an equation. (pizzas) Equations
- 12. Recall the prices for the pizzas from Pizza Grande. Each pizza is $8 and there is a $10 delivery charge. Hence if we want x pizzas delivered, then the total cost is given by the expression “8x + 10”. If we order x = 100 pizzas, then the cost is 8(100) + 10 = $810. Equations Expressions calculate future results. Now if we know the cost is $810 but forgot how many pizzas were ordered, we may backtrack to find x: 8x + 10 = 810 8x = 800 –10 –10 subtract the delivery cost, so the pizzas cost $800, divide this by $8/per pizza, x = 100 8 8 “8x + 10 = 810” is called an equation. Equations are set up to backtrack to the original input x or x’s, i.e. we want to solve equations. (pizzas)
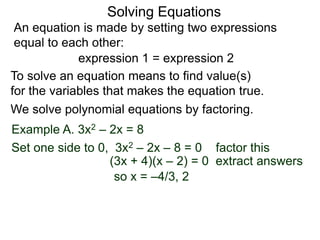
- 14. An equation is made by setting two expressions equal to each other: Solving Equations expression 1 = expression 2
- 15. An equation is made by setting two expressions equal to each other: Solving Equations expression 1 = expression 2 To solve an equation means to find value(s) for the variables that makes the equation true.
- 16. An equation is made by setting two expressions equal to each other: Solving Equations Example A. 3x2 – 2x = 8 We solve polynomial equations by factoring. expression 1 = expression 2 To solve an equation means to find value(s) for the variables that makes the equation true.
- 17. An equation is made by setting two expressions equal to each other: Solving Equations Example A. 3x2 – 2x = 8 We solve polynomial equations by factoring. Set one side to 0, 3x2 – 2x – 8 = 0 expression 1 = expression 2 To solve an equation means to find value(s) for the variables that makes the equation true.
- 18. An equation is made by setting two expressions equal to each other: Solving Equations Example A. 3x2 – 2x = 8 We solve polynomial equations by factoring. Set one side to 0, 3x2 – 2x – 8 = 0 factor this (3x + 4)(x – 2) = 0 expression 1 = expression 2 To solve an equation means to find value(s) for the variables that makes the equation true.
- 19. An equation is made by setting two expressions equal to each other: Solving Equations Example A. 3x2 – 2x = 8 We solve polynomial equations by factoring. Set one side to 0, 3x2 – 2x – 8 = 0 factor this (3x + 4)(x – 2) = 0 extract answers so x = –4/3, 2 expression 1 = expression 2 To solve an equation means to find value(s) for the variables that makes the equation true.
- 20. An equation is made by setting two expressions equal to each other: Solving Equations Example A. 3x2 – 2x = 8 We solve polynomial equations by factoring. Set one side to 0, 3x2 – 2x – 8 = 0 factor this (3x + 4)(x – 2) = 0 extract answers so x = –4/3, 2 expression 1 = expression 2 To solve an equation means to find value(s) for the variables that makes the equation true. To solve other equations such as rational equations, or equations with radicals, we have to transform them into polynomial equations.
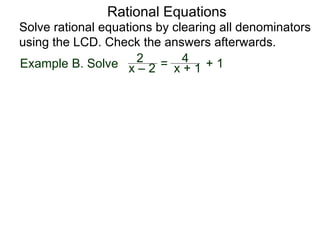
- 21. Rational Equations Solve rational equations by clearing all denominators using the LCD.
- 22. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards.
- 23. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve x – 2 2 = x + 1 4 + 1
- 24. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: x – 2 2 = x + 1 4 + 1
- 25. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: p (x – 2)(x + 1) * [ ] x – 2 2 = x + 1 4 + 1 x – 2 2 = x + 1 4 + 1
- 26. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] x – 2 2 = x + 1 4 + 1 (x + 1) x – 2 2 = x + 1 4 + 1
- 27. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] x – 2 2 = x + 1 4 + 1 (x + 1) (x – 2) x – 2 2 = x + 1 4 + 1
- 28. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] x – 2 2 = x + 1 4 + 1 (x + 1) (x – 2) (x + 1)(x – 2) x – 2 2 = x + 1 4 + 1
- 29. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] 2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2) x – 2 2 = x + 1 4 + 1 (x + 1) (x – 2) (x + 1)(x – 2) x – 2 2 = x + 1 4 + 1
- 30. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] 2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2) 2x + 2 = 4x – 8 + x2 – x – 2 x – 2 2 = x + 1 4 + 1 (x + 1) (x – 2) (x + 1)(x – 2) x – 2 2 = x + 1 4 + 1
- 31. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] 2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2) 2x + 2 = 4x – 8 + x2 – x – 2 2x + 2 = x2 + 3x – 10 x – 2 2 = x + 1 4 + 1 (x + 1) (x – 2) (x + 1)(x – 2) x – 2 2 = x + 1 4 + 1
- 32. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] 2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2) 2x + 2 = 4x – 8 + x2 – x – 2 2x + 2 = x2 + 3x – 10 0 = x2 + x – 12 x – 2 2 = x + 1 4 + 1 (x + 1) (x – 2) (x + 1)(x – 2) x – 2 2 = x + 1 4 + 1
- 33. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] 2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2) 2x + 2 = 4x – 8 + x2 – x – 2 2x + 2 = x2 + 3x – 10 0 = x2 + x – 12 0 = (x + 4)(x – 3) x = -4, 3 x – 2 2 = x + 1 4 + 1 (x + 1) (x – 2) (x + 1)(x – 2) x – 2 2 = x + 1 4 + 1
- 34. Rational Equations Solve rational equations by clearing all denominators using the LCD. Check the answers afterwards. Example B. Solve LCD = (x – 2)(x + 1), multiply the LCD to both sides of the equation: (x – 2)(x + 1) * [ ] 2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2) 2x + 2 = 4x – 8 + x2 – x – 2 2x + 2 = x2 + 3x – 10 0 = x2 + x – 12 0 = (x + 4)(x – 3) x = -4, 3 Both are good. x – 2 2 = x + 1 4 + 1 (x + 1) (x – 2) (x + 1)(x – 2) x – 2 2 = x + 1 4 + 1
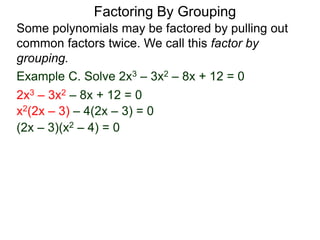
- 35. Factoring By Grouping Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping.
- 36. Example C. Solve 2x3 – 3x2 – 8x + 12 = 0 Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping. Factoring By Grouping
- 37. Example C. Solve 2x3 – 3x2 – 8x + 12 = 0 Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping. 2x3 – 3x2 – 8x + 12 = 0 Factoring By Grouping
- 38. Example C. Solve 2x3 – 3x2 – 8x + 12 = 0 Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping. 2x3 – 3x2 – 8x + 12 = 0 x2(2x – 3) – 4(2x – 3) = 0 Factoring By Grouping
- 39. Example C. Solve 2x3 – 3x2 – 8x + 12 = 0 Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping. 2x3 – 3x2 – 8x + 12 = 0 x2(2x – 3) – 4(2x – 3) = 0 (2x – 3)(x2 – 4) = 0 Factoring By Grouping
- 40. Example C. Solve 2x3 – 3x2 – 8x + 12 = 0 Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping. 2x3 – 3x2 – 8x + 12 = 0 x2(2x – 3) – 4(2x – 3) = 0 (2x – 3)(x2 – 4) = 0 (2x – 3)(x – 2)(x + 2) = 0 Factoring By Grouping
- 41. Example C. Solve 2x3 – 3x2 – 8x + 12 = 0 Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping. So x = 3/2, 2, –2 2x3 – 3x2 – 8x + 12 = 0 x2(2x – 3) – 4(2x – 3) = 0 (2x – 3)(x2 – 4) = 0 (2x – 3)(x – 2)(x + 2) = 0 Factoring By Grouping
- 42. Example C. Solve 2x3 – 3x2 – 8x + 12 = 0 Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping. So x = 3/2, 2, –2 2x3 – 3x2 – 8x + 12 = 0 x2(2x – 3) – 4(2x – 3) = 0 (2x – 3)(x2 – 4) = 0 (2x – 3)(x – 2)(x + 2) = 0 Except for some special cases, polynomial equations with degree 3 or more are solved with computers. Factoring By Grouping
- 43. Example C. Solve 2x3 – 3x2 – 8x + 12 = 0 Some polynomials may be factored by pulling out common factors twice. We call this factor by grouping. So x = 3/2, 2, –2 2x3 – 3x2 – 8x + 12 = 0 x2(2x – 3) – 4(2x – 3) = 0 (2x – 3)(x2 – 4) = 0 (2x – 3)(x – 2)(x + 2) = 0 We may also use the quadratic formula to solve all 2nd degree polynomial equations. Except for some special cases, polynomial equations with degree 3 or more are solved with computers. Factoring By Grouping
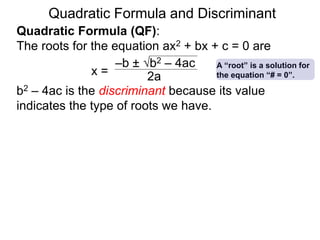
- 44. Quadratic Formula and Discriminant Quadratic Formula (QF):
- 45. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are x = –b ± b2 – 4ac 2a A “root” is a solution for the equation “# = 0”.
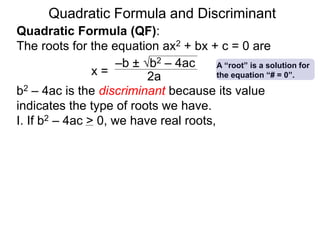
- 46. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are b2 – 4ac is the discriminant because its value indicates the type of roots we have. x = –b ± b2 – 4ac 2a A “root” is a solution for the equation “# = 0”.
- 47. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are b2 – 4ac is the discriminant because its value indicates the type of roots we have. I. If b2 – 4ac > 0, we have real roots, x = –b ± b2 – 4ac 2a A “root” is a solution for the equation “# = 0”.
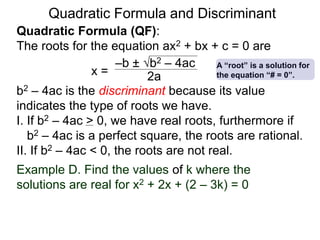
- 48. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are b2 – 4ac is the discriminant because its value indicates the type of roots we have. I. If b2 – 4ac > 0, we have real roots, furthermore if b2 – 4ac is a perfect square, the roots are rational. x = –b ± b2 – 4ac 2a A “root” is a solution for the equation “# = 0”.
- 49. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are b2 – 4ac is the discriminant because its value indicates the type of roots we have. I. If b2 – 4ac > 0, we have real roots, furthermore if b2 – 4ac is a perfect square, the roots are rational. II. If b2 – 4ac < 0, the roots are not real. x = –b ± b2 – 4ac 2a A “root” is a solution for the equation “# = 0”.
- 50. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are b2 – 4ac is the discriminant because its value indicates the type of roots we have. I. If b2 – 4ac > 0, we have real roots, furthermore if b2 – 4ac is a perfect square, the roots are rational. II. If b2 – 4ac < 0, the roots are not real. x = –b ± b2 – 4ac 2a Example D. Find the values of k where the solutions are real for x2 + 2x + (2 – 3k) = 0 A “root” is a solution for the equation “# = 0”.
- 51. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are b2 – 4ac is the discriminant because its value indicates the type of roots we have. I. If b2 – 4ac > 0, we have real roots, furthermore if b2 – 4ac is a perfect square, the roots are rational. II. If b2 – 4ac < 0, the roots are not real. x = –b ± b2 – 4ac 2a Example D. Find the values of k where the solutions are real for x2 + 2x + (2 – 3k) = 0 We need b2 – 4ac > 0, A “root” is a solution for the equation “# = 0”.
- 52. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are b2 – 4ac is the discriminant because its value indicates the type of roots we have. I. If b2 – 4ac > 0, we have real roots, furthermore if b2 – 4ac is a perfect square, the roots are rational. II. If b2 – 4ac < 0, the roots are not real. x = –b ± b2 – 4ac 2a Example D. Find the values of k where the solutions are real for x2 + 2x + (2 – 3k) = 0 We need b2 – 4ac > 0, i.e 4 – 4(2 – 3k) > 0. A “root” is a solution for the equation “# = 0”.
- 53. Quadratic Formula and Discriminant Quadratic Formula (QF): The roots for the equation ax2 + bx + c = 0 are b2 – 4ac is the discriminant because its value indicates the type of roots we have. I. If b2 – 4ac > 0, we have real roots, furthermore if b2 – 4ac is a perfect square, the roots are rational. II. If b2 – 4ac < 0, the roots are not real. x = –b ± b2 – 4ac 2a Example D. Find the values of k where the solutions are real for x2 + 2x + (2 – 3k) = 0 We need b2 – 4ac > 0, i.e 4 – 4(2 – 3k) > 0. – 4 + 12k > 0 or k > 1/3 A “root” is a solution for the equation “# = 0”.
- 54. Power Equations Equations of the Form xp/q = c
- 55. Power Equations The solution to the equation x 3 = –8 Equations of the Form xp/q = c
- 56. Power Equations The solution to the equation x 3 = –8 is x = √–8 3 Equations of the Form xp/q = c
- 57. Power Equations The solution to the equation x 3 = –8 is x = √–8 = –2. 3 Equations of the Form xp/q = c
- 58. Power Equations The solution to the equation x 3 = –8 is x = √–8 = –2. 3 Using fractional exponents, we write these steps as if x3 = –8 Equations of the Form xp/q = c
- 59. Power Equations The solution to the equation x 3 = –8 is x = √–8 = –2. 3 Using fractional exponents, we write these steps as if x3 = –8 then The reciprocal of the power 3 Equations of the Form xp/q = c x = (–8)1/3 = –2
- 60. Power Equations The solution to the equation x 3 = –8 is x = √–8 = –2. 3 Using fractional exponents, we write these steps as if x3 = –8 then The reciprocal of the power 3 Equations of the Form xp/q = c x = (–8)1/3 = –2 Rational power equations are equations of the type xR = c where R = p/q is a rational number.
- 61. Power Equations The solution to the equation x 3 = –8 is x = √–8 = –2. 3 Using fractional exponents, we write these steps as if x3 = –8 then To solve a power equation, take the reciprocal power, The reciprocal of the power 3 Equations of the Form xp/q = c x = (–8)1/3 = –2 Rational power equations are equations of the type xR = c where R = p/q is a rational number.
- 62. Power Equations The solution to the equation x 3 = –8 is x = √–8 = –2. 3 Using fractional exponents, we write these steps as if x3 = –8 then To solve a power equation, take the reciprocal power, so if xR = c, The reciprocal of the power 3 xp/q = c Equations of the Form xp/q = c x = (–8)1/3 = –2 Rational power equations are equations of the type xR = c where R = p/q is a rational number.
- 63. Power Equations The solution to the equation x 3 = –8 is x = √–8 = –2. 3 Using fractional exponents, we write these steps as if x3 = –8 then To solve a power equation, take the reciprocal power, so if xR = c, then x = (±)c1/R The reciprocal of the power 3 xp/q = c Equations of the Form xp/q = c x = (–8)1/3 = –2 Rational power equations are equations of the type xR = c where R = p/q is a rational number. Reciprocate the powers
- 64. Power Equations The solution to the equation x 3 = –8 is x = √–8 = –2. 3 Using fractional exponents, we write these steps as if x3 = –8 then To solve a power equation, take the reciprocal power, so if xR = c, then x = (±)c1/R Reciprocate the powers The reciprocal of the power 3 xp/q = c x = (±)cq/p or Equations of the Form xp/q = c x = (–8)1/3 = –2 Rational power equations are equations of the type xR = c where R = p/q is a rational number.
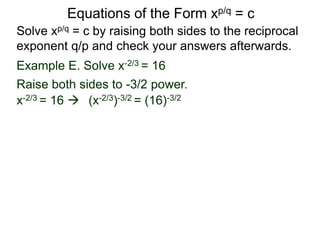
- 65. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards.
- 66. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16
- 67. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 Raise both sides to -3/2 power.
- 68. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 Raise both sides to -3/2 power.
- 69. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 Raise both sides to -3/2 power. x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64 We check that –1/64 is also a solution.
- 70. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 Example F. Solve (2x – 3)3/2 = -8 Raise both sides to -3/2 power. x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64 We check that –1/64 is also a solution.
- 71. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 Example F. Solve (2x – 3)3/2 = -8 Raise both sides to -3/2 power. Raise both sides to 2/3 power. x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64 We check that –1/64 is also a solution.
- 72. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 Example F. Solve (2x – 3)3/2 = -8 Raise both sides to -3/2 power. Raise both sides to 2/3 power. (2x – 3)3/2 = -8 [(2x – 3)3/2]2/3 = (-8)2/3 x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64 We check that –1/64 is also a solution.
- 73. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 Example F. Solve (2x – 3)3/2 = -8 Raise both sides to -3/2 power. Raise both sides to 2/3 power. (2x – 3)3/2 = -8 [(2x – 3)3/2]2/3 = (-8)2/3 (2x – 3) = 4 x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64 We check that –1/64 is also a solution.
- 74. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 Example F. Solve (2x – 3)3/2 = -8 Raise both sides to -3/2 power. Raise both sides to 2/3 power. (2x – 3)3/2 = -8 [(2x – 3)3/2]2/3 = (-8)2/3 (2x – 3) = 4 2x = 7 x = 7/2 x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64 We check that –1/64 is also a solution.
- 75. Equations of the Form xp/q = c Solve xp/q = c by raising both sides to the reciprocal exponent q/p and check your answers afterwards. Example E. Solve x-2/3 = 16 x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64 We check that –1/64 is also a solution. Example F. Solve (2x – 3)3/2 = -8 Raise both sides to -3/2 power. Raise both sides to 2/3 power. (2x – 3)3/2 = -8 [(2x – 3)3/2]2/3 = (-8)2/3 (2x – 3) = 4 2x = 7 x = 7/2 Since x = 7/2 doesn't work because 43/2 = -8, there is no solution.
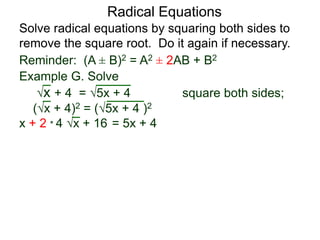
- 77. Radical Equations Solve radical equations by squaring both sides to remove the square root.
- 78. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2
- 79. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4
- 80. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2
- 81. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2 x + 2 * 4 x + 16 = 5x + 4
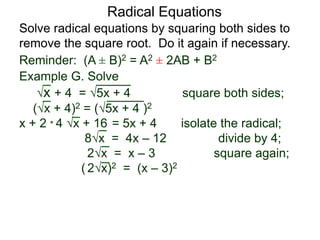
- 82. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2 x + 2 * 4 x + 16 = 5x + 4 isolate the radical; 8x = 4x – 12
- 83. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2 x + 2 * 4 x + 16 = 5x + 4 isolate the radical; 8x = 4x – 12 divide by 4; 2x = x – 3
- 84. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2 x + 2 * 4 x + 16 = 5x + 4 isolate the radical; 8x = 4x – 12 divide by 4; 2x = x – 3 square again; ( 2x)2 = (x – 3)2
- 85. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2 x + 2 * 4 x + 16 = 5x + 4 isolate the radical; 8x = 4x – 12 divide by 4; 2x = x – 3 square again; ( 2x)2 = (x – 3)2 4x = x2 – 6x + 9
- 86. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2 x + 2 * 4 x + 16 = 5x + 4 isolate the radical; 8x = 4x – 12 divide by 4; 2x = x – 3 square again; ( 2x)2 = (x – 3)2 4x = x2 – 6x + 9 0 = x2 – 10x + 9
- 87. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2 x + 2 * 4 x + 16 = 5x + 4 isolate the radical; 8x = 4x – 12 divide by 4; 2x = x – 3 square again; ( 2x)2 = (x – 3)2 4x = x2 – 6x + 9 0 = x2 – 10x + 9 0 = (x – 9)(x – 1) x = 9, x = 1
- 88. Radical Equations Solve radical equations by squaring both sides to remove the square root. Do it again if necessary. Reminder: (A ± B)2 = A2 ± 2AB + B2 Example G. Solve x + 4 = 5x + 4 square both sides; (x + 4)2 = (5x + 4 )2 x + 2 * 4 x + 16 = 5x + 4 isolate the radical; 8x = 4x – 12 divide by 4; 2x = x – 3 square again; ( 2x)2 = (x – 3)2 4x = x2 – 6x + 9 0 = x2 – 10x + 9 0 = (x – 9)(x – 1) x = 9, x = 1 Only 9 is good.
- 89. The absolute value of x is the distance measured from x to 0 and it is denoted as |x|. Absolute Value Equations
- 90. The absolute value of x is the distance measured from x to 0 and it is denoted as |x|. Because it is distance, |x| is nonnegative. Absolute Value Equations
- 91. The absolute value of x is the distance measured from x to 0 and it is denoted as |x|. Because it is distance, |x| is nonnegative. Absolute Value Equations Algebraic definition of absolute value: |x|= x if x is positive or 0. –x (opposite of x) if x is negative. {
- 92. The absolute value of x is the distance measured from x to 0 and it is denoted as |x|. Because it is distance, |x| is nonnegative. Absolute Value Equations Algebraic definition of absolute value: |x|= x if x is positive or 0. –x (opposite of x) if x is negative. { Hence | -5 | = –(-5) = 5.
- 93. The absolute value of x is the distance measured from x to 0 and it is denoted as |x|. Because it is distance, |x| is nonnegative. Absolute Value Equations Algebraic definition of absolute value: |x|= x if x is positive or 0. –x (opposite of x) if x is negative. { Hence | -5 | = –(-5) = 5. Since the absolute value is never negative, an equation such as |x4 – 3x + 1 | = – 2 doesn't have any solution.
- 94. The absolute value of x is the distance measured from x to 0 and it is denoted as |x|. Because it is distance, |x| is nonnegative. Absolute Value Equations Algebraic definition of absolute value: |x|= x if x is positive or 0. –x (opposite of x) if x is negative. { Hence | -5 | = –(-5) = 5. Since the absolute value is never negative, an equation such as |x4 – 3x + 1 | = – 2 doesn't have any solution. Fact I. |x*y| = |x|*|y|. For example, |-2*3 | = |-2|*|3| = 6.
- 95. The absolute value of x is the distance measured from x to 0 and it is denoted as |x|. Because it is distance, |x| is nonnegative. Absolute Value Equations Algebraic definition of absolute value: |x|= x if x is positive or 0. –x (opposite of x) if x is negative. { Hence | -5 | = –(-5) = 5. Since the absolute value is never negative, an equation such as |x4 – 3x + 1 | = – 2 doesn't have any solution. Fact I. |x*y| = |x|*|y|. For example, |-2*3 | = |-2|*|3| = 6. Warning: In general |x ± y| |x| ± |y|.
- 96. The absolute value of x is the distance measured from x to 0 and it is denoted as |x|. Because it is distance, |x| is nonnegative. Absolute Value Equations Algebraic definition of absolute value: |x|= x if x is positive or 0. –x (opposite of x) if x is negative. { Hence | -5 | = –(-5) = 5. Since the absolute value is never negative, an equation such as |x4 – 3x + 1 | = – 2 doesn't have any solution. Fact I. |x*y| = |x|*|y|. For example, |-2*3 | = |-2|*|3| = 6. Warning: In general |x ± y| |x| ± |y|. For instance, | 2 – 3 | |2| – |3| |2| + |3|.
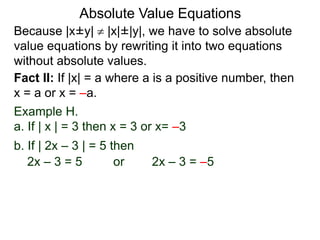
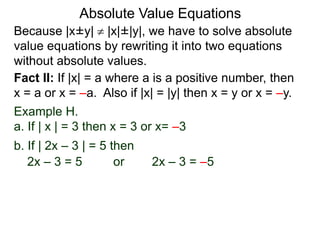
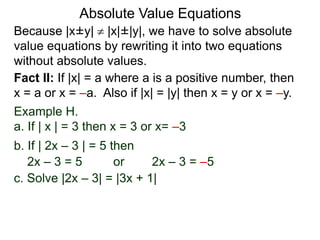
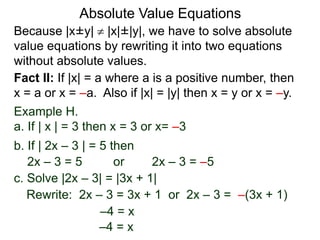
- 97. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations
- 98. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a.
- 99. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Example H. a. If | x | = 3
- 100. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Example H. a. If | x | = 3 then x = 3 or x= –3
- 101. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Example H. a. If | x | = 3 then x = 3 or x= –3 b. If | 2x – 3 | = 5 then
- 102. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Example H. a. If | x | = 3 then x = 3 or x= –3 b. If | 2x – 3 | = 5 then 2x – 3 = 5 or 2x – 3 = –5
- 103. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Also if |x| = |y| then x = y or x = –y. Example H. a. If | x | = 3 then x = 3 or x= –3 b. If | 2x – 3 | = 5 then 2x – 3 = 5 or 2x – 3 = –5
- 104. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Also if |x| = |y| then x = y or x = –y. Example H. a. If | x | = 3 then x = 3 or x= –3 b. If | 2x – 3 | = 5 then 2x – 3 = 5 or 2x – 3 = –5 c. Solve |2x – 3| = |3x + 1|
- 105. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Also if |x| = |y| then x = y or x = –y. Example H. a. If | x | = 3 then x = 3 or x= –3 b. If | 2x – 3 | = 5 then 2x – 3 = 5 or 2x – 3 = –5 c. Solve |2x – 3| = |3x + 1| Rewrite: 2x – 3 = 3x + 1 or 2x – 3 = –(3x + 1)
- 106. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Also if |x| = |y| then x = y or x = –y. Example H. a. If | x | = 3 then x = 3 or x= –3 b. If | 2x – 3 | = 5 then 2x – 3 = 5 or 2x – 3 = –5 c. Solve |2x – 3| = |3x + 1| Rewrite: 2x – 3 = 3x + 1 or 2x – 3 = –(3x + 1) –4 = x –4 = x
- 107. Because |x±y| |x|±|y|, we have to solve absolute value equations by rewriting it into two equations without absolute values. Absolute Value Equations Fact II: If |x| = a where a is a positive number, then x = a or x = –a. Also if |x| = |y| then x = y or x = –y. Example H. a. If | x | = 3 then x = 3 or x= –3 b. If | 2x – 3 | = 5 then 2x – 3 = 5 or 2x – 3 = –5 c. Solve |2x – 3| = |3x + 1| Rewrite: 2x – 3 = 3x + 1 or 2x – 3 = –(3x + 1) –4 = x or 2x – 3 = –3x – 1 –4 = x or x = 2/5
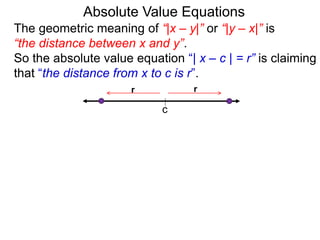
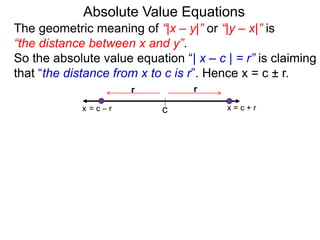
- 108. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. Absolute Value Equations
- 109. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. So the absolute value equation “| x – c | = r” is claiming that “the distance from x to c is r”. Absolute Value Equations
- 110. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. So the absolute value equation “| x – c | = r” is claiming that “the distance from x to c is r”. Absolute Value Equations c
- 111. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. So the absolute value equation “| x – c | = r” is claiming that “the distance from x to c is r”. Absolute Value Equations c r r
- 112. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. So the absolute value equation “| x – c | = r” is claiming that “the distance from x to c is r”. Hence x = c ± r. Absolute Value Equations c r x = c + r r x = c – r
- 113. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. So the absolute value equation “| x – c | = r” is claiming that “the distance from x to c is r”. Hence x = c ± r. Absolute Value Equations Example G. Draw and solve for x geometrically if |x – 7| = 12. c r x = c + r r x = c – r
- 114. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. So the absolute value equation “| x – c | = r” is claiming that “the distance from x to c is r”. Hence x = c ± r. Absolute Value Equations Example G. Draw and solve for x geometrically if |x – 7| = 12. The equation asks for the locations of x's that are 12 units away from 7. c r x = c + r r x = c – r
- 115. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. So the absolute value equation “| x – c | = r” is claiming that “the distance from x to c is r”. Hence x = c ± r. Absolute Value Equations Example G. Draw and solve for x geometrically if |x – 7| = 12. The equation asks for the locations of x's that are 12 units away from 7. c r x = c + r 7 12 12 r x = c – r
- 116. The geometric meaning of “|x – y|” or “|y – x|” is “the distance between x and y”. So the absolute value equation “| x – c | = r” is claiming that “the distance from x to c is r”. Hence x = c ± r. Absolute Value Equations Example G. Draw and solve for x geometrically if |x – 7| = 12. The equation asks for the locations of x's that are 12 units away from 7. c r x = c + r So to the right x = 7 + 12 = 19. and to the left x = 7 – 12 = – 5, 7 12 12 x = 19 x = – 5 r x = c – r
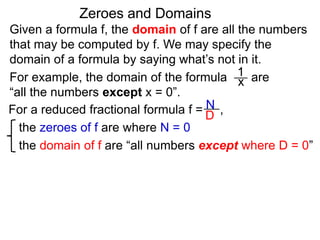
- 117. Given a formula f, the domain of f are all the numbers that may be computed by f. Zeroes and Domains
- 118. Given a formula f, the domain of f are all the numbers that may be computed by f. We may specify the domain of a formula by saying what’s not in it. Zeroes and Domains
- 119. Given a formula f, the domain of f are all the numbers that may be computed by f. We may specify the domain of a formula by saying what’s not in it. For example, the domain of the formula are “all the numbers except x = 0”. Zeroes and Domains 1 x
- 120. Given a formula f, the domain of f are all the numbers that may be computed by f. We may specify the domain of a formula by saying what’s not in it. For example, the domain of the formula are “all the numbers except x = 0”. Zeroes and Domains 1 x For a reduced fractional formula f = , the zeroes of f are where N = 0 the domain of f are “all numbers except where D = 0” N D
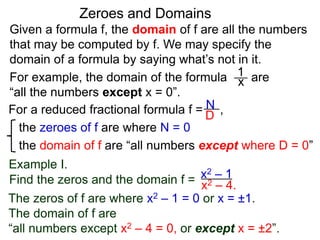
- 121. Given a formula f, the domain of f are all the numbers that may be computed by f. We may specify the domain of a formula by saying what’s not in it. For example, the domain of the formula are “all the numbers except x = 0”. Zeroes and Domains Example I. 1 x For a reduced fractional formula f = , the zeroes of f are where N = 0 the domain of f are “all numbers except where D = 0” N D x2 – 4. Find the zeros and the domain f = x2 – 1
- 122. Given a formula f, the domain of f are all the numbers that may be computed by f. We may specify the domain of a formula by saying what’s not in it. For example, the domain of the formula are “all the numbers except x = 0”. Zeroes and Domains Example I. 1 x For a reduced fractional formula f = , the zeroes of f are where N = 0 the domain of f are “all numbers except where D = 0” N D x2 – 4. Find the zeros and the domain f = x2 – 1 The zeros of f are where x2 – 1 = 0 or x = ±1.
- 123. Given a formula f, the domain of f are all the numbers that may be computed by f. We may specify the domain of a formula by saying what’s not in it. For example, the domain of the formula are “all the numbers except x = 0”. Zeroes and Domains Example I. 1 x For a reduced fractional formula f = , the zeroes of f are where N = 0 the domain of f are “all numbers except where D = 0” N D x2 – 4. Find the zeros and the domain f = x2 – 1 The zeros of f are where x2 – 1 = 0 or x = ±1. The domain of f are “all numbers except x2 – 4 = 0, or except x = ±2”.
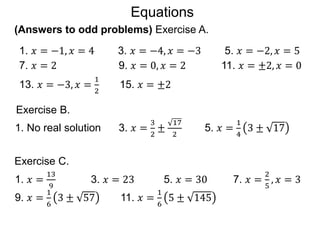
- 124. A. Solve the following equations by factoring. 5. x2 – 3x = 10 9. x3 – 2x2 = 0 6. x2 = 4 7. 2x(x – 3) + 4 = 2x – 4 10. 2x2(x – 3) = –4x 8. x(x – 3) + x + 6 = 2x2 + 3x 1. x2 – 3x – 4 = 0 2. x2 – 2x – 15 = 0 3. x2 + 7x + 12 = 0 4. –x2 – 2x + 8 = 0 11. 4x2 = x4 12. 7x2 = –4x3 – 3x 13. 5 = (x + 2)(2x + 1) 14. (x + 1)2 = x2 + (x – 1)2 15. (x + 3)2 – (x + 2)2 = (x + 1)2 B. Solve the following equations by the quadratic formula. If the answers are not real numbers, just state so. 1. x2 – x + 1 = 0 2. x2 – x – 1 = 0 3. x2 – 3x – 2 = 0 4. x2 – 2x + 3 = 0 5. 2x2 – 3x – 1 = 0 6. 3x2 = 2x + 3 Equations
- 125. Rational Equations 7. 1 x + 1 x – 1 = 5 6 8. 1 x + 1 x + 2 = 3 4 9. 2 x + 1 x + 1 = 3 2 10. + 5 x + 2 = 2 2 x – 1 11. – 1 x + 1 = 3 2 12. 6 x + 2 – 4 x + 1 = 1 1 x – 2 x 6 3 1 2 3 5 2 3 – + = x 1. x 4 6 –3 1 8 –5 – 1 – = x 2. x 4 5 3 2 10 7 4 3 + – = x 3. x 8 12 –5 7 16 –5 + 1 + = x (x – 20) = x – 3 100 30 100 20 5. (x + 5) – 3 = (x – 5) 100 25 100 20 6. C. Clear the denominators of the equations, then solve.
- 126. Radical Equations and Power Equations D. Isolate one radical if needed, square both sides, do it again if necessary, to solve for x. Check your answers. 1. x – 2 = x – 4 2. x + 3 = x + 1 3. 2x – 1 = x + 5 4. 4x + 1 – x + 2 = 1 5. x – 2 = x + 3 – 1 6. 3x + 4 = 3 – x – 1 7. 2x + 5 = x + 4 8. 5 – 4x – 3 – x = 1 E. Solve by raising both sides to an appropriate power. No calculator. 1. x –2 = 1/4 2. x –1/2 = 1/4 3. x –3 = –8 4. x –1/3 = –8 5. x –2/3 = 4 6. x –3/2 = 8 7. x –2/3 = 1/4 8. x –3/2 = – 1/8 9. x 1.5 = 1/27 10. x 1.25 = 32 11. x –1.5 = 27 12. x –1. 25 = 1/32
- 127. F. Solve for x. 1. Is it always true that I+x| = x? Give reason for your answer. 2. Is it always true that |–x| = x? Give reason for your answer. Absolute Value Equations 3. |4 – 5x| = 3 4. |3 + 2x| = 7 5. |–2x + 3| = 5 6. |4 – 5x| = –3 7. |2x + 1| – 1= 5 8. 3|2x + 1| – 1= 5 9. |4 – 5x| = |3 + 2x| 11. |4 – 5x| = |2x + 1| 12. |3x + 1| = |5 – x| 10. |–2x + 3|= |3 – 2x| Solve geometrically for x. Draw the solution. 13. |x – 2| = 1 14. |3 + x| = 5 15. | –9 + x| = –7 x – 4 G. Find the zeros and the domain of the following rational formulas. 2x – 1 1. x2 – 1. x2 – 4 3. 5x + 7 2. 3x + 5 x2 – x x2 – x – 2 4. x2 – 4x 5. x2 + x – 2 x2 + 2x 6. x2 + x + 2 2x2 – x – 1 7. x3 + 2x x4 – 4x 8. x3 – 8 16. |2 + x| = 1 17. |3 – x| = –5 18. | –9 – x| = 8
- 128. (Answers to odd problems) Exercise A. Exercise B. 1. No real solution 3. 𝑥 = 3 2 ± 17 2 5. 𝑥 = 1 4 3 ± 17 1. 𝑥 = −1, 𝑥 = 4 3. 𝑥 = −4, 𝑥 = −3 5. 𝑥 = −2, 𝑥 = 5 7. 𝑥 = 2 9. 𝑥 = 0, 𝑥 = 2 11. 𝑥 = ±2, 𝑥 = 0 13. 𝑥 = −3, 𝑥 = 1 2 15. 𝑥 = ±2 1. 𝑥 = 13 9 3. 𝑥 = 23 5. 𝑥 = 30 7. 𝑥 = 2 5 , 𝑥 = 3 9. 𝑥 = 1 6 3 ± 57 11. 𝑥 = 1 6 5 ± 145 Exercise C. Equations
- 129. Exercise D. 1. 𝑥 = 4 3. 𝑥 = 4 5. 𝑥 = 6 7. 𝑥 = 4, 𝑥 = 4 9 Exercise E. 1. 𝑥 = ±2 3. 𝑥 = 2 2 3 5. 𝑥 = 1 8 7. 𝑥 = 8 9. 𝑥 = 1 9 11. 𝑥 = 1 9 1. If 𝑥 < 0, the statement is not true 3. 𝑥 = 1 5 , 𝑥 = 7/5 5. 𝑥 = −1, 𝑥 = 4 7. 𝑥 = 5/2, 𝑥 = −7/2 9. 𝑥 = 1/7, 𝑥 = 7/3 11. 𝑥 = 3 7 , 𝑥 = 5 3 13. |𝑥 − 2| = 1 𝑥 = 1 or 𝑥 = 3 Exercise F. 2 1 3 left 1 right 1 Radical Equations and Power Equations
- 130. 15. No solution 17. No solution Exercise G. 1. Zeros: 𝑥 = 1/2, domain: 𝑥 ≠ 4 3. Zeros: 𝑥 = ±2, domain: 𝑥 ≠ ±4 5. Zeros: 𝑥 = 0, 𝑥 = 4, domain: 𝑥 ≠ −2, 𝑥 ≠ 1 7. Zeros: 𝑥 = − 1 2 , 𝑥 = 1, domain: 𝑥 ≠ 0 Absolute Value Equations






















![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
p
(x – 2)(x + 1) * [ ]
x – 2
2 =
x + 1
4
+ 1
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-25-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
x – 2
2 =
x + 1
4
+ 1
(x + 1)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-26-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
x – 2
2 =
x + 1
4
+ 1
(x + 1) (x – 2)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-27-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
x – 2
2 =
x + 1
4
+ 1
(x + 1) (x – 2) (x + 1)(x – 2)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-28-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2)
x – 2
2 =
x + 1
4
+ 1
(x + 1) (x – 2) (x + 1)(x – 2)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-29-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2)
2x + 2 = 4x – 8 + x2 – x – 2
x – 2
2 =
x + 1
4
+ 1
(x + 1) (x – 2) (x + 1)(x – 2)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-30-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2)
2x + 2 = 4x – 8 + x2 – x – 2
2x + 2 = x2 + 3x – 10
x – 2
2 =
x + 1
4
+ 1
(x + 1) (x – 2) (x + 1)(x – 2)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-31-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2)
2x + 2 = 4x – 8 + x2 – x – 2
2x + 2 = x2 + 3x – 10
0 = x2 + x – 12
x – 2
2 =
x + 1
4
+ 1
(x + 1) (x – 2) (x + 1)(x – 2)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-32-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2)
2x + 2 = 4x – 8 + x2 – x – 2
2x + 2 = x2 + 3x – 10
0 = x2 + x – 12
0 = (x + 4)(x – 3) x = -4, 3
x – 2
2 =
x + 1
4
+ 1
(x + 1) (x – 2) (x + 1)(x – 2)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-33-320.jpg)
![Rational Equations
Solve rational equations by clearing all denominators
using the LCD. Check the answers afterwards.
Example B. Solve
LCD = (x – 2)(x + 1), multiply the LCD to both sides
of the equation:
(x – 2)(x + 1) * [ ]
2(x + 1) = 4(x – 2) + 1*(x + 1)(x – 2)
2x + 2 = 4x – 8 + x2 – x – 2
2x + 2 = x2 + 3x – 10
0 = x2 + x – 12
0 = (x + 4)(x – 3) x = -4, 3
Both are good.
x – 2
2 =
x + 1
4
+ 1
(x + 1) (x – 2) (x + 1)(x – 2)
x – 2
2 = x + 1
4 + 1](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-34-320.jpg)
































![Equations of the Form xp/q = c
Solve xp/q = c by raising both sides to the reciprocal
exponent q/p and check your answers afterwards.
Example E. Solve x-2/3 = 16
Example F. Solve (2x – 3)3/2 = -8
Raise both sides to -3/2 power.
Raise both sides to 2/3 power.
(2x – 3)3/2 = -8 [(2x – 3)3/2]2/3 = (-8)2/3
x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64
We check that –1/64 is also a solution.](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-72-320.jpg)
![Equations of the Form xp/q = c
Solve xp/q = c by raising both sides to the reciprocal
exponent q/p and check your answers afterwards.
Example E. Solve x-2/3 = 16
Example F. Solve (2x – 3)3/2 = -8
Raise both sides to -3/2 power.
Raise both sides to 2/3 power.
(2x – 3)3/2 = -8 [(2x – 3)3/2]2/3 = (-8)2/3
(2x – 3) = 4
x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64
We check that –1/64 is also a solution.](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-73-320.jpg)
![Equations of the Form xp/q = c
Solve xp/q = c by raising both sides to the reciprocal
exponent q/p and check your answers afterwards.
Example E. Solve x-2/3 = 16
Example F. Solve (2x – 3)3/2 = -8
Raise both sides to -3/2 power.
Raise both sides to 2/3 power.
(2x – 3)3/2 = -8 [(2x – 3)3/2]2/3 = (-8)2/3
(2x – 3) = 4
2x = 7 x = 7/2
x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64
We check that –1/64 is also a solution.](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-74-320.jpg)
![Equations of the Form xp/q = c
Solve xp/q = c by raising both sides to the reciprocal
exponent q/p and check your answers afterwards.
Example E. Solve x-2/3 = 16
x-2/3 = 16 (x-2/3)-3/2 = (16)-3/2 = 1/64
We check that –1/64 is also a solution.
Example F. Solve (2x – 3)3/2 = -8
Raise both sides to -3/2 power.
Raise both sides to 2/3 power.
(2x – 3)3/2 = -8 [(2x – 3)3/2]2/3 = (-8)2/3
(2x – 3) = 4
2x = 7 x = 7/2
Since x = 7/2 doesn't work because 43/2 = -8,
there is no solution.](https://image.slidesharecdn.com/1-210617190943/85/1-3-solving-equations-y-75-320.jpg)