Congruent
In geometry, congruent means identical in shape and size. Congruence can be applied to line segments, angles, and figures. Any two line segments are said to be congruent if they are equal in length. Two angles are said to be congruent if they are of equal measure. Two triangles are said to be congruent if their corresponding sides and angles are equal. Let us learn more about congruence and congruent figures in this article.
| 1. | Congruent Meaning in Geometry |
| 2. | Congruent Figures |
| 3. | Congruent and Similar Figures |
| 4. | FAQs on Congruent |
Congruent Meaning in Geometry
The word 'congruent' means 'exactly equal' in terms of shape and size. Even when we turn, flip, or rotate the shapes, they remain equal. For example, draw two circles of the same radius, then cut them out and place them on one another. We will notice that they will superimpose each other, that is, they will be placed completely over each other. This shows that the two circles are congruent. The following circles are said to be congruent since they have an equal radius, and they can be placed exactly over one another. The symbol that is used to show the congruence of figures is "≅". Since circle A is congruent to circle B, we can express this fact as follows: Circle A ≅ Circle B.

Congruent Figures
The congruence of any two figures can be seen if they can be placed exactly over each other. The word 'congruence' is used to express the relationship of two figures that are said to be congruent. In other words, if any two geometrical figures can be superimposed on each other, they are termed as congruent figures. This property applies to all figures like triangles, quadrilaterals, and so on. Apart from figures, line segments and angles are also termed as congruent if they are of equal measure. Observe the following figure to understand what congruent figures mean.
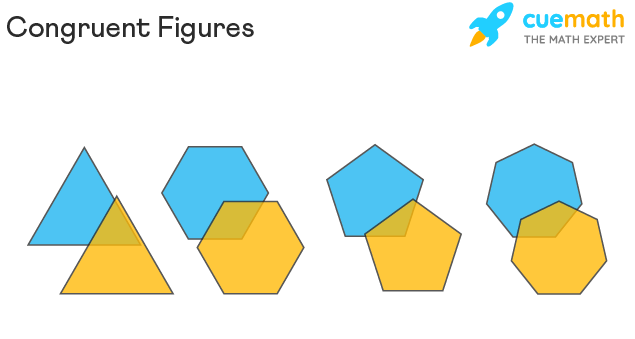
Congruent and Similar Figures
There is a difference between congruent and similar figures. Congruent figures have the same corresponding side lengths and the corresponding angles are of equal measure. However, similar figures may have the same shape, but their size may not be the same.
For example, observe the following triangles which show the difference between congruent and similar figures. In the congruent figures, we can see that all the corresponding sides and angles are of equal measure. However, if we notice the similar figures, we see that the corresponding angles are of equal measure, but the sides are not of equal length.
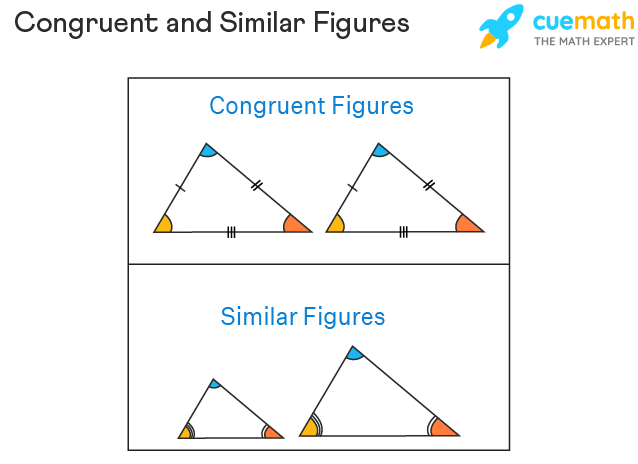
Congruence of Triangles
Two triangles are said to be congruent if their sides are equal in length, the angles are of equal measure, and they can be superimposed on each other.

In the figure given above, Δ ABC and Δ PQR are congruent triangles. This means that the corresponding angles and corresponding sides in both the triangles are equal.
Sides: AB = PQ, BC = QR and AC = PR;
Angles: ∠A = ∠P, ∠B = ∠Q, and ∠C = ∠R.
Therefore, Δ ABC ≅ Δ PQR
The following are the congruence theorems or the triangle congruence criteria that help to prove the congruence of triangles.
- SSS (Side, Side, Side)
- SAS (side, angle, side)
- ASA (angle, side, angle)
- AAS (angle, angle, side)
- RHS (Right angle-Hypotenuse-Side or the Hypotenuse Leg theorem)
Related Links
Check out the following pages related to congruence and congruent figures.
Congruent Examples
-
Example 1: The two quadrilaterals shown below are congruent. Which angle in quadrilateral PQRS corresponds to ∠WXY in quadrilateral WXYZ?

Solution:
Let us identify the corresponding parts in both the quadrilaterals.
∠WXY is marked with four arcs in quadrilateral WXYZ.
∠QRS is also marked with four arcs in quadrilateral PQRS. This shows that they are equal, which means ∠QRS coincides with ∠WXY. Therefore, ∠WXY corresponds to ∠QRS. -
Example 2: Emma has four squares with the following side lengths: Square A, side = 5 inches, Square B, side = 7 inches, Square C, side = 5 inches, Square D, side = 8 inches. She wants two squares that can be placed exactly one over the other. Can you help her choose the congruent squares?
Solution: Squares with the same sides will superimpose on each other because they will be congruent. So, Emma should find two squares whose side lengths are exactly the same. In the given list, we can see that Square A and Square C have sides of the same length, that is, 5 inches. Therefore, Emma can choose Square A and C because they can be placed exactly one over the other.

FAQs on Congruent
What are Congruent Figures?
Congruent figures are those which have the sides of the same length and angles of the same measure. In other words, when one figure superimposes the other, the figures are termed as congruent figures. They fit on each other exactly even when they are rotated or flipped.
How to Prove that Triangles are Congruent?
Two triangles are said to be congruent if their corresponding sides are equal in length, and their corresponding angles are equal in measure.
What are the Properties of Congruence?
The properties of congruence are applicable to lines, angles, and figures. They can be listed as follows: Reflexive property, Symmetric property, and Transitive property.
- The reflexive property of congruence says that a line segment, an angle, or a shape is always congruent to itself. For example, ∠P≅∠P
- The symmetric property says that if one figure is congruent to another, then the second one is also congruent to the first. For any two angles P and Q, if ∠P ≅∠Q, then ∠Q ≅∠P.
- The transitive property of congruence states that if line 1 is congruent to line 2, and line 2 is congruent to line 3, then line 1 is also congruent to line 3.
What Shape has all Congruent Sides?
The square is the only shape in which all the sides are congruent and all the angles are of equal measure.
What are the 5 Triangle Congruence Criteria?
The following list shows the triangle congruence criteria or the theorems that prove the congruence of triangles.
- SSS (Side, Side, Side)
- SAS (Side, Angle, Side)
- ASA (Angle, Side, Angle)
- AAS (Angle, Angle, Side)
- RHS (Right angle-Hypotenuse-Side or the Hypotenuse Leg Theorem)
Are Vertical Angles Always Congruent?
Yes, vertical angles are always congruent because according to the vertical angles theorem, when two straight lines intersect each other, the opposite angles that are formed are always equal (congruent). These angles are called vertically opposite angles or vertical angles.
What is Another Word for Congruent?
Congruent means 'identical' in shape and size. Congruent shapes are also called coinciding shapes. These shapes can superimpose each other and can fit exactly one over the other.
What makes the Angles Congruent?
Angles are said to be congruent if their measures are exactly the same in degrees or radians. If ∠P = ∠Q, then both the angles are said to be congruent angles.
visual curriculum