Lecture 29 Review Frequency response examples Frequency response
















- Slides: 27

Lecture 29 • Review: • Frequency response examples • Frequency response plots & signal spectra • Filters • Related educational modules: –Section 2. 8. 1, 2. 8. 2

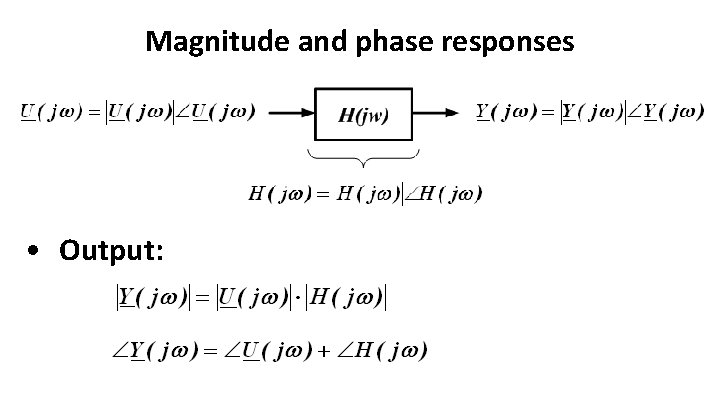
Frequency Response • Systems are characterized in the frequency domain, by their frequency response, H(j ) • Magnitude response: the ratio of the output amplitude to the input amplitude as a function of frequency • Phase response: the difference between the output phase and the input phase, as a function of frequency

Magnitude and phase responses • Output:

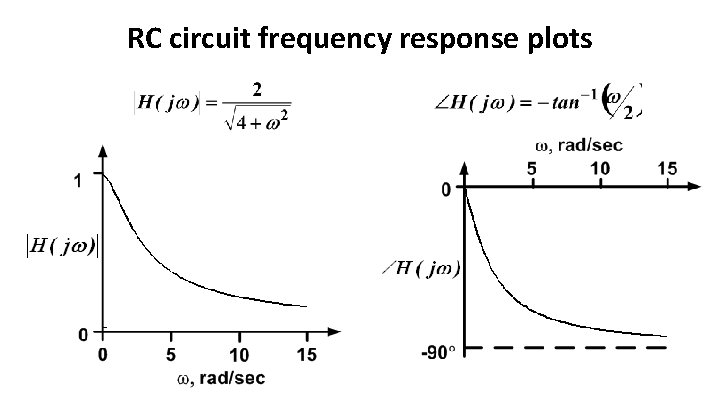
Review: RC circuit frequency response • Determine the magnitude and phase responses of the circuit below. vin(t) is the input and vout(t) is the output

• Annotate previous slide to denote |H|=mag resp, <H = phase resp.

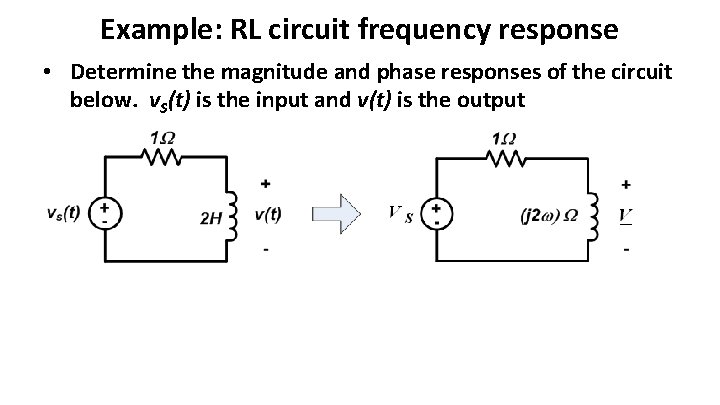
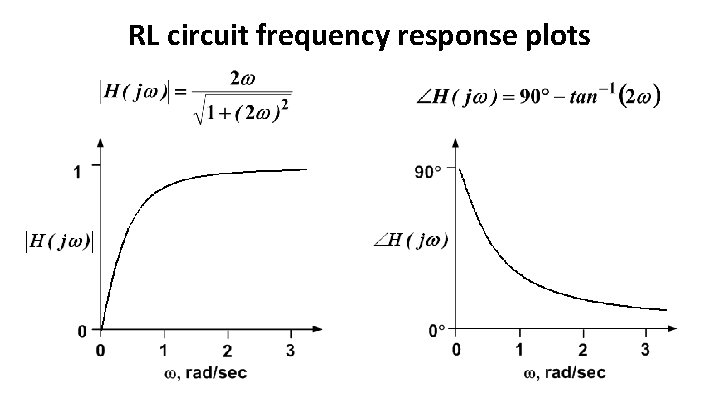
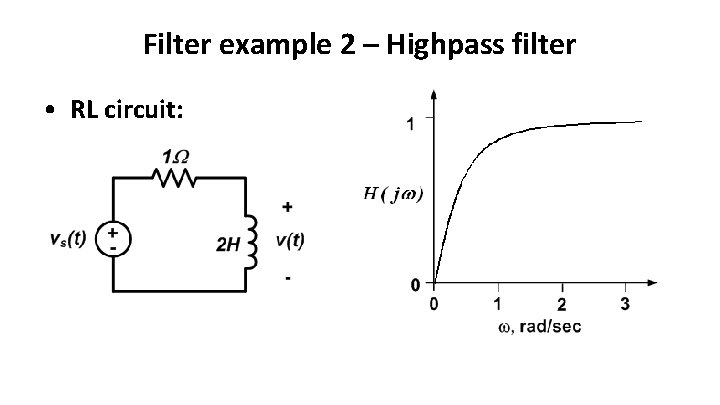
Example: RL circuit frequency response • Determine the magnitude and phase responses of the circuit below. v. S(t) is the input and v(t) is the output

Frequency response plots • Frequency responses are often presented graphically in the form of two plots: • Magnitude response vs. frequency • Phase response vs. frequency

RC circuit frequency response plots

RL circuit frequency response plots

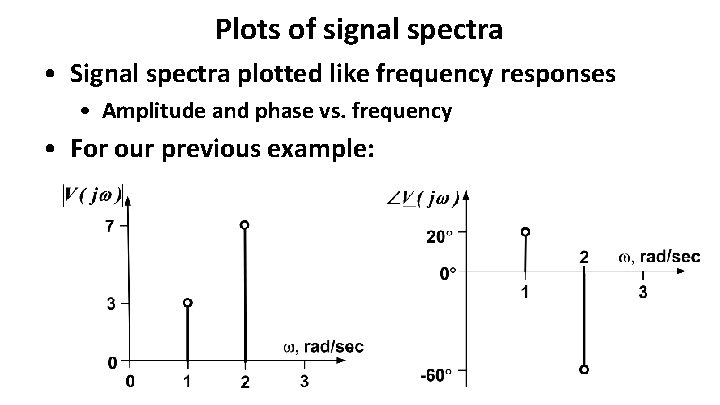
Signal spectra • The frequency domain content of a signal is called the spectrum of the signal • Example: v(t) = 3 cos(t+20 ) + 7 cos(2 t-60 ) • Spectrum:

Plots of signal spectra • Signal spectra plotted like frequency responses • Amplitude and phase vs. frequency • For our previous example:

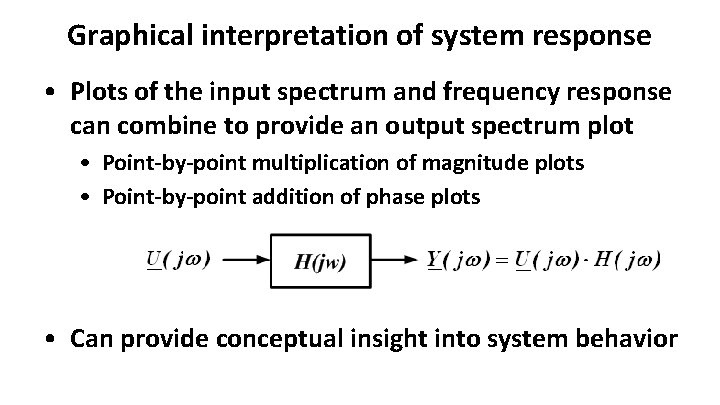
Graphical interpretation of system response • Plots of the input spectrum and frequency response can combine to provide an output spectrum plot • Point-by-point multiplication of magnitude plots • Point-by-point addition of phase plots • Can provide conceptual insight into system behavior

Example – RL circuit response to example input

Frequency selective circuits and filters • Circuits are often categorized by the general “shape” of their magnitude response • The response in some frequency ranges will be high relative to the input; these frequencies are passed • H(j ) is “large” in these frequency ranges • The response in some frequency ranges will be low relative to the input; these frequencies are stopped • H(j ) is “small” in these frequency ranges

Filters • Circuits which select certain frequency ranges to pass and other frequency ranges to stop are often called frequency selective circuits or filters • Example: audio system graphic equalizer • The range (or band) of frequencies that are passed by the filter is called the passband • The range (or band) of frequencies that are stopped by the filter is called the stopband

Specific case I – Lowpass filters • Lowpass filters pass low frequencies and stop high frequencies • The boundary between the two bands is the cutoff frequency, c • “Low” frequencies are less than c, “high” frequencies are greater than c

• On previous slide, note that IDEAL filters absolutely remove all components outside the passband. • Also point out that these cannot be implemented in the real world (turns out that they would need to respond to the input before the input is applied – they need to see into the future)

Specific case II – Highpass filters • Highpass filters pass high frequencies and stop low frequencies • The boundary between the two bands is (still) called the cutoff frequency, c

Additional filter categories • Filters are often categorized by the order of the differential equation governing the circuit • e. g. First order filter, second order filter • Filters can also be bandpass or bandstop • A band of frequencies between two cutoff frequencies is either passed or stopped • Lowpass & highpass filters can be first or higher order • Bandpass & bandstop filters must be at least second order • We will only work with first order filters in this course

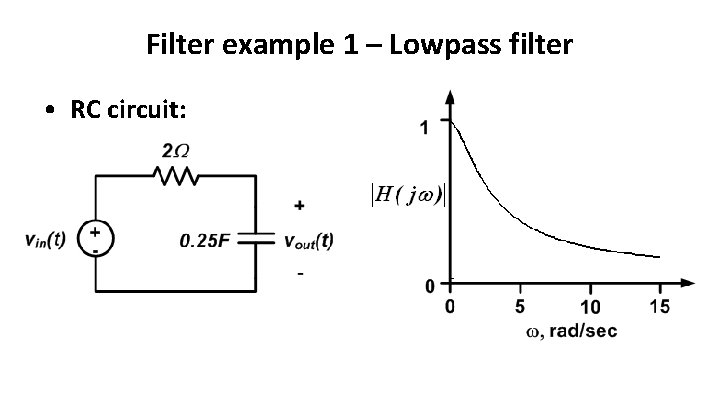
Filter example 1 – Lowpass filter • RC circuit:

Filter example 2 – Highpass filter • RL circuit:

Non-ideal first order filters • Realizable filters do not have sharp transitions between the passband stopband • So where is the cutoff frequency ( c)? • Define the cutoff frequency where the magnitude • response is times the maximum magnitude • Why? • The power is (generally) the square of the signal the cutoff frequency is where we have half of the maximum power (it is sometimes called the half power point)

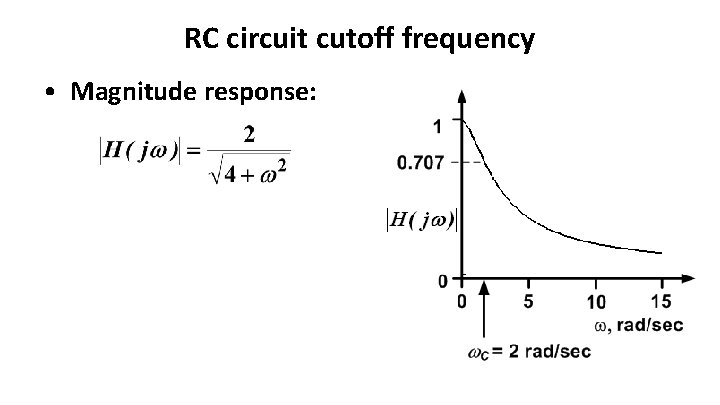
RC circuit cutoff frequency • Magnitude response:

• Annotate previous slide to calculate maximum value and frequency where we have 0. 707 times maximum value

RL circuit cutoff frequency • Magnitude response:

• Annotate to show calculation of cutoff frequency

 01:640:244 lecture notes - lecture 15: plat, idah, farad
01:640:244 lecture notes - lecture 15: plat, idah, farad Marginal relative frequency
Marginal relative frequency Frequency vs relative frequency
Frequency vs relative frequency Peak factor formula
Peak factor formula Vmax= aw
Vmax= aw Relative frequency bar chart
Relative frequency bar chart Joint frequency vs marginal frequency
Joint frequency vs marginal frequency Conditional relative frequency example
Conditional relative frequency example Cut off frequency formula
Cut off frequency formula Multistage amplifier circuit
Multistage amplifier circuit Inductor frequency response
Inductor frequency response Bjt frequency response
Bjt frequency response Frequency response methods and stability
Frequency response methods and stability Analog electronics
Analog electronics Formula for cutoff frequency
Formula for cutoff frequency Tanggapan frekuensi tinggi penguat transistor bipolar
Tanggapan frekuensi tinggi penguat transistor bipolar Frequency response of feedback amplifier
Frequency response of feedback amplifier Source follower frequency response
Source follower frequency response Frequency response cbe
Frequency response cbe Delay line canceller in radar
Delay line canceller in radar Frequency response
Frequency response Natural response and forced response
Natural response and forced response What is natural response
What is natural response Primary immune response and secondary immune response
Primary immune response and secondary immune response Review response letter
Review response letter Chapter review motion part a vocabulary review answer key
Chapter review motion part a vocabulary review answer key Writ of certiorari ap gov example
Writ of certiorari ap gov example Narrative review vs systematic review
Narrative review vs systematic review